Vijay Kumar Bhat – Diameter and girth of tensor product of zero-divisor graphs

SMVD University, India
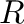
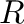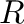Let $R$ be a commutative ring with identity $1\neq 0$. Zero-divisor graph of $R$ is a graph with vertex set as non-zero zero-divisors of 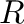$R$ such that distinct vertices $x$ and $y$ are adjacent if $x.y = 0$.

Consider the rings $R=\mathbb{Z}_{m}$ and $S= \mathbb{Z}_{n}$, $G$ and $H$ their zero-divisor graphs respectively with $\mid G \mid , \mid H \mid \geq 2$. We discuss the diameter and girth of tensor product and lexicographic product of $G$ and $H$.

This is joint work with Pradeep Singh.