
 to
to
 for a squarefree number
for a squarefree number 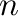 . We show that the lattice of all clones on the squarefree set
. We show that the lattice of all clones on the squarefree set
 which contain the addition of
which contain the addition of
 is finite. We provide an upper bound for the cardinality of this lattice through an injective function to the direct product of the lattices of all
is finite. We provide an upper bound for the cardinality of this lattice through an injective function to the direct product of the lattices of all
 -linearly closed clonoids,
-linearly closed clonoids,
 , to the
, to the  power, where
power, where
 . Furthermore, we prove that these clones can be generated by a set of functions of arity at most
. Furthermore, we prove that these clones can be generated by a set of functions of arity at most
 .
.