Arkady Tsurkov – IBN-varieties of algebras

Federal University Of Rio Grande Do Norte, Brazil
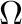
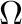We consider in a class of all universal algebras of a signature $\Omega$ some variety $\Theta $. We denote by $F_{\Theta }\left( X\right) $ the free algebra of this variety generated by the set of free generators $X$. We consider only finitely generated free algebras. We denote by $\left\vert X\right\vert $ the quantity of the elements of the set $X$.

Definition 1   We say that the variety $\Theta $ is an IBN-variety (or variety which has an IBN propriety), if from $F_{\Theta }\left( X\right) \cong F_{\Theta }\left( Y\right) $ we can conclude that $\left\vert X\right\vert =\left\vert Y\right\vert $.

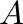The concept of variety with IBN (invariant basic number) propriety first appeared in ring theory. It is known that if we consider some field $k$, the vector space $V$ over this field such that $\dim _{k}V=\aleph _{0}$ ring $A$ of all linear operators on this vector space ( $A=\mathrm{End}_{k}V$), then $% A\cong A\oplus A\cong A\oplus A\oplus A\cong \ldots \cong \underset{n\text{ }% times}{\underbrace{A\oplus \ldots \oplus A}}\cong \ldots $, i.e., the variety $_{A}\mathfrak{M}$ of all rights modules over the ring $A$ 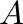has not the IBN propriety.

The proving of the IBN propriety of some variety is very important in universal algebraic geometry. This is a milestone in the study of the relation between geometric and automorphic equivalences of algebras of this variety.

We will look at some examples where the IBN property of certain varieties can be proved directly. For example for every signature 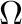$\Omega$ the variety defined by the empty set of identities has the IBN property.

We will discuss very simple but very useful

Theorem 1   If $\Delta ,\Theta $ some varieties of universal algebras of a signature $% \Omega $, $\Delta \subset \Theta $ and $\Delta $ is an IBN-variety then $% \Theta $ is also an IBN-variety.

We will consider applications of this theorem.

We will consider many-sorted universal algebras as well as one-sorted. So all concepts and all results will by generalized for the many-sorted case.