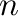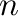Anatolii V. Zhuchok – The least dimonoid congruence on the free $n$-nilpotent $g$-dimonoid

Luhansk Taras Shevchenko National University (Starobilsk, Ukraine)
The $g$-dimonoids [2] are a generalization of dimonoids [1] while every 0-dialgebra with associative operations [3] is a linear analog of a $g$-dimonoid. Free 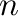$n$-nilpotent $g$-dimonoids were constructed in [4].

Let $X$ be an arbitrary nonempty set, and let $w$ be an arbitrary word over $X$. For every $x\in X$, the number of occurrences of the element $x$ in $w$ is denoted by $d_{x}(w)$. If $\rho $ is a congruence on a $g$-dimonoid $(D,\dashv,\vdash)$ such that $(D,\dashv,\vdash)/\rho $ is a dimonoid, we say that $\rho $ is a dimonoid congruence. If $\rho $ is a congruence on a $g$-dimonoid $(D,\dashv,\vdash)$ such that operations of $(D,\dashv,\vdash)/\rho $ coincide, we say that $\rho $ is a semigroup congruence.

Theorem. Let $G_{n}(X)$ be the free 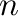$n$-nilpotent $g$-dimonoid, $\omega_{1}, \omega_{2} \in G_{n}(X)$.

(i) Define a relation $\widetilde{\kappa}$ on $G_{n}(X)$ by

$\omega_{1} \widetilde{\kappa} \omega_{2}$ if and only if one of the following conditions holds:

(1) $\omega_{1}=(w_{1} ,u_{1}), \omega_{2}=(w_{2} ,u_{2}) \in G_{n} \backslash \{0\}$ and $w_{1}=w_{2}$, $d_b (u_1)=d_b (u_2)$;

(2) $\omega_{1}=\omega_{2}=0$.

Then $\widetilde{\kappa}$ is the least dimonoid congruence on $G_{n}(X)$.

(ii) Define a relation $\widetilde{\beta}$ on $G_{n}(X)$ by

$\omega_{1} \widetilde{\beta} \omega_{2}$ if and only if one of the following conditions holds:

(1) $\omega_{1}=(w_{1} ,u_{1}), \omega_{2}=(w_{2} ,u_{2}) \in G_{n} \backslash \{0\}$ and $w_{1}=w_{2}$;

(2) $\omega_{1}=\omega_{2}=0$.

Then $\widetilde{\beta}$ is the least semigroup congruence on $G_{n}(X)$.


References

[1]
Loday, J.-L.: Dialgebras. In: Dialgebras and related operads: Lect. Notes Math., vol. 1763, Berlin: Springer-Verlag, 7–66 (2001)
[2]
Movsisyan, Y., Davidov, S., Safaryan, M.: Construction of free $g$-dimonoids. Algebra Discrete Math. 18, no. 1, 138–148 (2014)
[3]
Pozhidaev, A.P.: 0-dialgebras with bar-unity and nonassociative Rota-Baxter algebras. Sib. Math. J. 50, no. 6, 1070–1080 (2009)
[4]
Zhuchok, Yul. V.: On one class of algebras. Algebra Discrete Math. 18, no. 2, 306–320 (2014)